lightingpolew.blogg.se

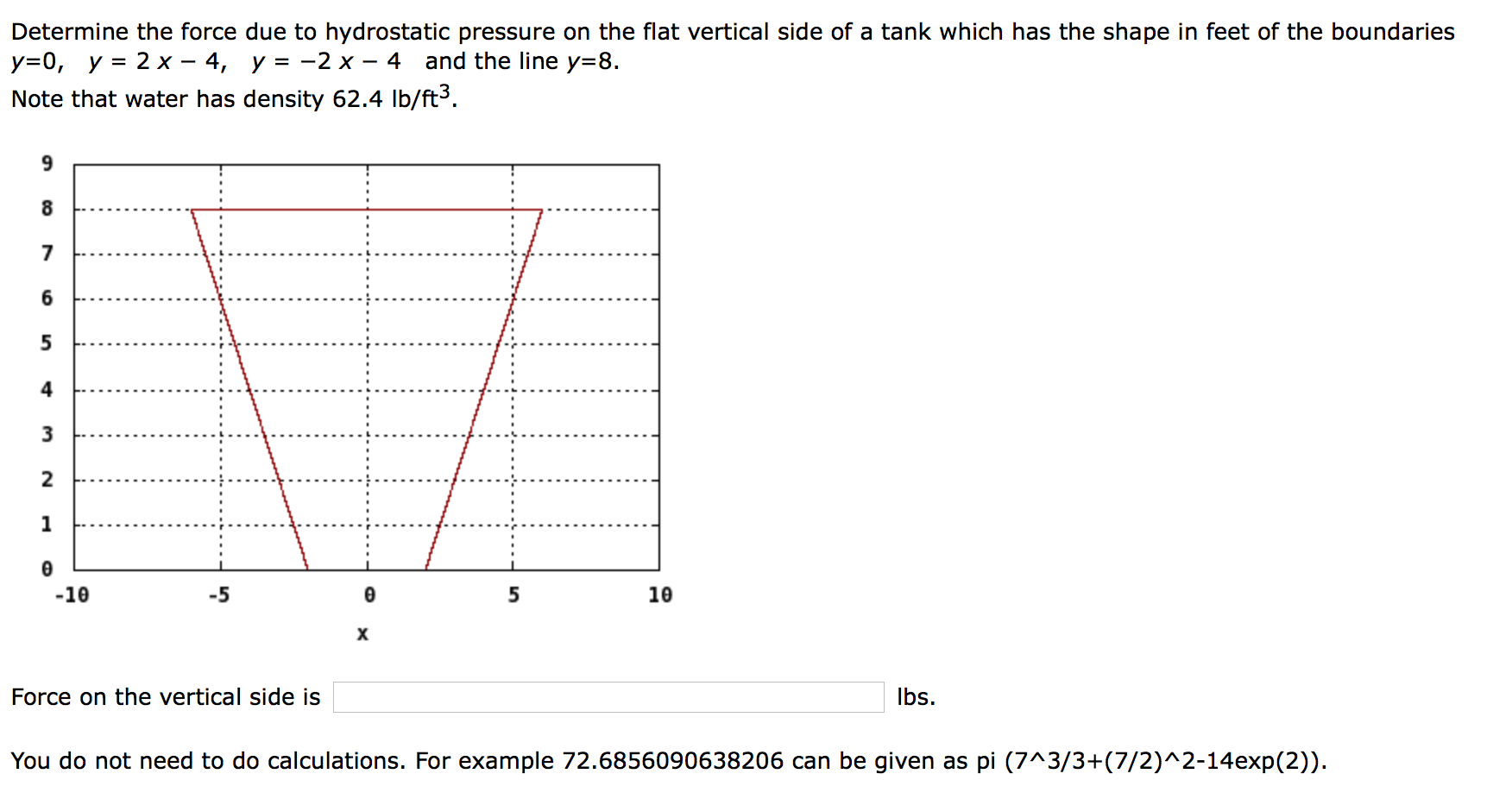
Using Technology and Tables to Evaluate Integrals.The Second Fundamental Theorem of Calculus.Constructing Accurate Graphs of Antiderivatives.Determining Distance Traveled from Velocity.Using Derivatives to Describe Families of Functions.Using Derivatives to Identify Extreme Values.Derivatives of Functions Given Implicitly.Derivatives of Other Trigonometric Functions.Interpreting, Estimating, and Using the Derivative.The Derivative of a Function at a Point.The same computation also applies to show how hydrostatic pressure results in a buoyant force on a submerged object equaling the weight of the displaced fluid. This shows that, no matter the shape of the container, hydrostatic pressure will always result in the container's applying a total force precisely cancelling the weight of its contents.

You can also add the constant atmospheric pressure to the integrand if you like, and the result will not change. Similar computations indicate that $F_x, \, F_y$ are zero. More generally, at each point on the tank surface, call it $S$, the tank pushes into the water in the direction normal to $S$ with a pressure $\rho g(h-z)$, where $z$ is the vertical distance of the point in question from the bottom of the tank. In one realization of your example, the diagram in parsa639's answer indicates how this causes the discrepancy: the container pushes down on the water where its horizontal cross-sections thin from area $A$ to $A/2$, so the upward force from the bottom of the tank must be larger than the water weight to cancel both this and gravity. These are different statements because hydrostatic pressure is being applied to the entire surface of the tank (and hence the entire surface is pushing back by Newton's $3$rd law), so the force at the bottom is not the only thing coming into play in the force balance. The point is that the net vertical force on the water must be zero, which is distinct from requiring that the force from the tank bottom be equal to gravity. This might seem surprising, however, because we intuitively expect the tank to only need to feel the force necessary to support the water's weight- so where's the disconnect? As others have discussed, the computations are computing different things, but only the second approach yields the total force on the bottom of the tank.